Fundamental geometric principles are required to determine the value of x in polygons that are similar. The sides and angles of similar polygons are proportional and congruent with one another. Because of this inherent relationship, ratios between adjacent sides or angles can be calculated, revealing previously unknown values like x.
Calculating x using the polygons’ known dimensions or angles likewise in query, in exercises 5-8, the polygons are similar. find the value of x. (see example 2.), becomes possible through systematic comparison and proportional reasoning. This cycle highlights the utility of mathematical likeness in tackling obscure factors inside polygons that work with exact numerical examination and critical thinking in different settings.
Is it Possible to Find the Value of x in Similar Polygons?
Congruent angles and proportional sides are found in polygons that are similar to one another. Considering this corresponding relationship, it is feasible to track down the worth of x in comparative polygons using proportions. One can determine the value by establishing and solving proportions based on the known corresponding sides or angles, x as a function of the scale factor that separates the polygons.
The application of geometric principles to the problem of determining the values of unknown variables like x makes it easier to analyze and solve mathematical problems in a variety of situations involving polygons that are similar.
Most Ideal Way to Find the Worth of x in Similar Polygons
The best method for tracking down the worth of x in comparable polygons is through Gauth, utilizing its high-level abilities in mathematical examination and relative thinking. Gauth makes use of the similarity principle, which states that similar polygons have sides and angles that are proportional to one another.
Students are able to accurately set up and solve proportions by utilizing Gauth’s interactive tools and entering the polygon’s dimensions or angles. Gauth’s natural connection point considers continuous changes and visual portrayals, making it the best device to work with an exhaustive comprehension of mathematical connections and to track down the exact worth of x in comparable polygons proficiently and really.
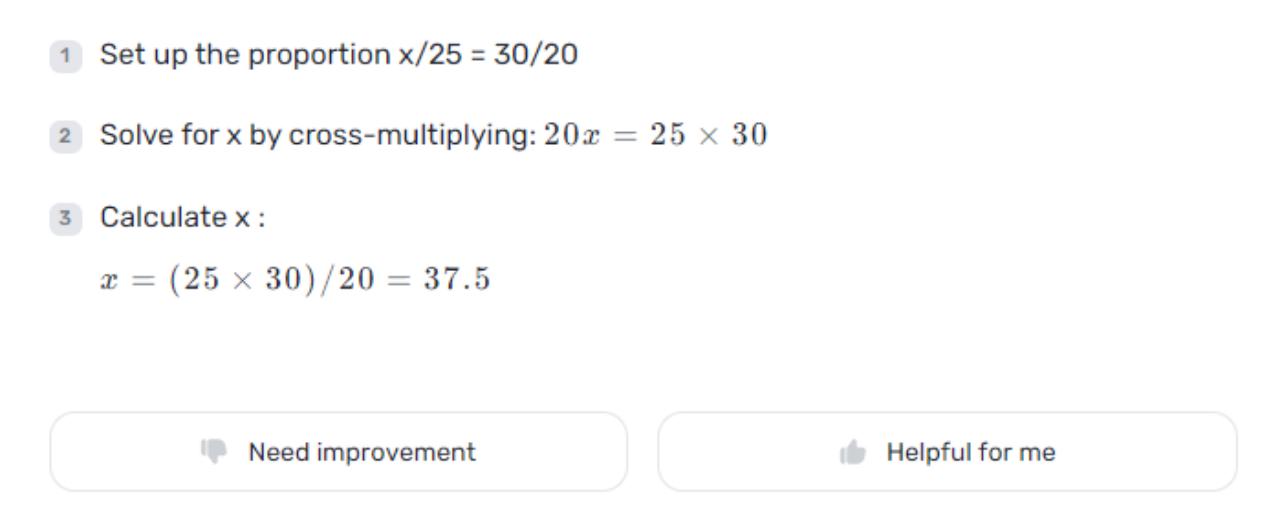
Steps For Finding The Value Of x
If you are interested in knowing the procedure of finding the value of x using Gauth. Then the following steps must be in mind.
Step 1: Access Gauth
Start by signing into Gauth and exploring to the math segment where mathematical estimations are performed. This segment gives devices explicitly intended to deal with mathematical figures and numerical activities connected with polygons.

Step 2: Confirm Similarity
Verify that the in-question polygons meet the similarity criteria. Ensure that the fundamental conditions for applying similarity principles are met by checking that corresponding sides and angles are proportional.
Step 3: Input Polygon Information
Enter the known aspects or points of one of the comparable polygons into Gauth’s intelligent devices. This step includes contributing explicit mathematical qualities that act as the beginning stage for setting up extents to tackle for x.
Step 4: Layout Extents
Use Gauth’s devices to set up extents in view of the similitude proportions between comparing sides or points of the comparative polygons. These proportions, which are essential for determining the value of the unknown variable, are mathematical representations of the relationships that exist between the corresponding parts of the polygons. x.
Step 5: Compute x
Solve the established proportions and determine the value by making use of Gauth’s computational capabilities. X. Gauth makes use of algorithms that compute a numerical solution for the given proportions. X based on the data that was entered and the similarity conditions that were established.
Step 6: Review and Interpret the Results
Following the computation, x basically assesses the determined worth inside the setting of the comparative polygons. Ensure that the calculated value accurately reflects the proportional relationship between the polygons based on their respective angles or dimensions by adhering to similarity principles. The computed value’s relevance and accuracy are confirmed in this verification step. X within the provided geometric context.
Conclusion
In polygons that are similar to one another, finding “x” is not impossible. The mysteries of these shapes can be deciphered with the help of Gauth’s theorem and the careful application of geometric principles. Let us embrace the curiosity and determination that propel us toward discovery as we continue to explore the depths of geometry.